私は構造の設計に関する研究をおこなっています。構造は、ミクロからマクロなスケールまであらゆるところに存在し、私たちの生活や環境に深く関わっています。建物や橋、さらには自然界に見られる様々な形状や構造物は、私たちの社会を支える重要な要素です。現在は、構造の中でも基本的なエッセンスであるふたつの要素「ちから」と「かたち」に着目しています。このふたつの要素は、構造物の性能や美しさを決定づける重要な要素であり、私の研究の中心的なテーマとなっています。これらの要素を研究するために必要なものは、幾何学、構造力学、数値計算などの理論的な知識です。これらの要素を歯車のように組み合わせて新しい構造を設計することで、従来の枠を超えた革新的な構造物を生み出すことを目指しています。
誰も見たことがない形状や現象を目指す
私のリサーチのモチベーションは、今まで誰も見たことがない形状や現象を実現したいという想いに根ざしています。私が目指すのは、200年後も淘汰されずに残るような、原点のような構造システムの実現です。このような構造物は、未来の建築やデザインに新たな可能性をもたらすと信じています。また、SFの父と呼ばれる小説家のジュール・ヴェルヌが語ったとされる「人間が想像できることは必ず実現できる」という言葉を信じており、妄想を現実にするビジョン・ドリブンな研究を心がけています。
構造の可能性
私の研究では、「こんな形状や力学特性を自在に作れたらすごいけど、誰もができるような簡単な方法でそれを作るのは難しい」と考えられる構造物を設計可能にする普遍的な手法を探求しています。
例えば、私が取り組んでいる「Gauss Challenge Project」は、一言でいえば“平面から多様な曲面をつくる” という研究です。数学者のカール・フリードリヒ・ガウスが「平面から曲面を生成する問題はチャレンジングな課題である」と語っていたことにちなんで名付けました。そのチャレンジングな課題について、工学的な手法を用いて実現することに挑戦しています。
曲面を大雑把に分類すると鞍型、平面・円筒、椀型の3タイプがあり、それぞれ2方向の山なりのカーブの上下の組合わせによって決まるガウス曲率という曲面上の曲率の正負が異なります。鞍型や平面・円筒の曲面は身の回りにある平面状の構造物を変形させれば簡単に生成できます。例えば、一枚の紙の端を丸めると円筒ができます。一方で椀型の曲面を材料の特性のみで生成することは難しく、負のポアソン比を持つ構造（オーゼティック構造）という機械的メタマテリアル（以下では、メタマテリアルとする）を用いれば生成できます。メタマテリアルのmetaはギリシャ語で、英語ではbeyond（超える）を意味します。つまり直訳すると「超越材料」といわれるもので、自然界には存在しない特殊な構造をもった材料あるいは構造物のことです。これらの構造物の力学特性は、材料の特性よりも、その構造を構成している内部形状の幾何的な特性によって特徴づけられます。例えば、凸な形状を持つ六角形が隙間なく並んだハニカム構造を一方向から圧縮すると直交方向にふくらみます。しかし、この六角形が蝶ネクタイのような凹な形状である場合、構造物はオーゼティック構造としての機能をもつようになり一方向に圧縮して縮めると、直交方向にも縮んで全体的に小さくなります。構造物の内部形状を操作してオーゼティック構造とそうでない構造の変形をうまく組み合わせることで、ありとあらゆる形の曲面をつくる方法を探求するのがこのプロジェクトの目的です。
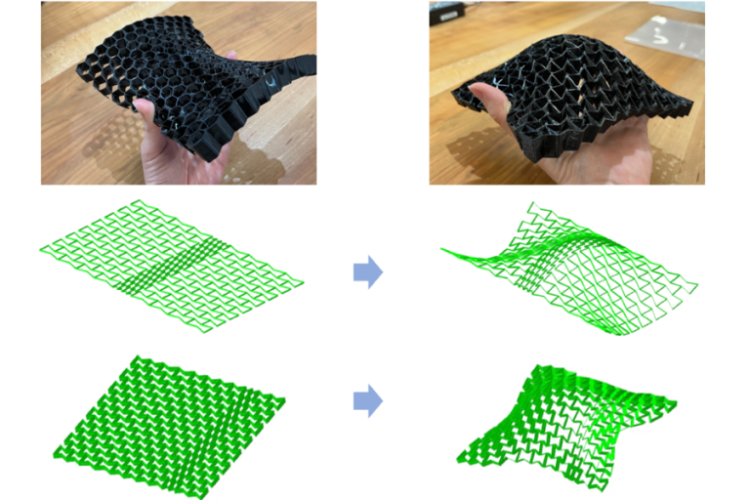
また、メタマテリアルのような特殊な変形機能を有する構造物のさらなる可能性として、将来的には構造物そのものを計算資源として使用する、物理的リザバーコンピューティングの分野への応用などが考えられると思っています。 これにより、電気ではなく構造の変形によって動作するコンピュータが開発されることがあるかもしれません。
今後の展開
以前は構造をコンピュータ上で設計して特性を解析して、それらを論文にまとめるという形式で研究を進めていました。最近は3Dプリンターを活用して、直感的に設計した構造をとりあえず作ってみて実際に触って特性を調べてから特性を解析するということが多くなりました。また、研究成果を論文で発表するだけでなく具体的なものとして紹介する機会も増えています。実際に成形した構造物を異分野の方々に見ていただくことで、思いもよらないアイデアや刺激をいただく事も多く、私の研究のモチベーションになっています。例えば、構造物の「ちから」や「かたち」のアプローチによる設計法を用いた音響制御やソフトロボティクスへの応用などに注目しています。さらに、変形することやその感触そのものを利用した知育玩具への展開の可能性などのアイデアは私にとって新しい発展の可能性でした。
私の研究が、単なる挑戦にとどまらず、その成果が建築だけでなく、実際の社会の様々なシーンで役立つ形で展開されると嬉しいと思っています。これにより、未来の建築だけでなく、デザイン、社会がどのように進化していくのか、非常に楽しみです。
My research centers on structural design. Structures exist everywhere, from micro to macro scales, and are deeply intertwined with our lives and environments. Buildings, bridges, and various forms of structures found in nature are importantl elements that support our society. Currently, my focus is on two fundamental elements of structure—“force” and “form”—which are the basic essence of structural design. These elements are critical in determining the performance and beauty of structures and are the central theme of my research. To study these elements, theoretical knowledge in geometry, structural mechanics, and numerical computation is essential. By combining these elements like gears, I aim to design innovative structures.
Aiming for Unseen Shapes and Phenomena
The motivation behind my research is rooted in the desire to realize shapes and phenomena that no one has ever seen before. My aim is to achieve a structural system that will still be in use 200 years from now. I believe that such structures will bring new possibilities to future architecture and design. I also believe in the words of the father of science fiction, Jules Verne, who said, "Anything one man can imagine, other men can make real." This philosophy drives my vision-driven research to turn imagination into reality.
The Possibilities of Structures
My research explores universal methods that make it possible to design structures that would be considered "amazing if we could freely create such shapes and mechanical properties, but it is difficult to create them in a simple way that anyone can do".
For example, the "Gauss Challenge Project" I am working on is, in short, a study of "creating versatile curved surfaces from a plane." It is named after the mathematician Carl Friedrich Gauss, who referred to the problem of generating curved surfaces from a plane as a "challenging task." I am taking on this challenging problem, attempting to realize it using engineering methods. Curved surfaces can be roughly classified into three types: saddle- like shape, plane/cylinder, and dome-like shape. These are determined by the combination of two directions of curvature, known as Gaussian curvature, which describes whether the curvature on the surface is positive or negative. Saddle-like shapes and plane/cylindrical surfaces can be easily generated by deforming flat structures. For example, rolling the edge of a sheet of paper will create a cylindrical shape. On the other hand, generating a dome-like surface is more difficult, but can be achieved using structures with a negative Poisson's ratio (auxetic structures), a type of mechanical metamaterial. The term "metamaterial" comes from the Greek word "meta," meaning "beyond." Metamaterials are materials or structures with special properties that do not exist in nature. The internal geometry of these structures rather than the properties of the materials characterize their mechanical properties. For example, when compressing a structure tiled with convex hexagonal honeycombs in one direction, it expands in the orthogonal direction. However, when compressing an auxetic structure with concave hexagonal honeycombs, it shrinks in the transverse direction. The goal of this project is to explore ways to create various types of curved surfaces by utilizing these special deformations: auxetic and non-auxetic.
Additionally, as a potential application of structures like metamaterials, I believe that in the future, structures could be used as computational resources, with possible applications in the field of physical reservoir computing. This may lead to the development of computers that operate not by electricity but by deformation of the structure.
Future Directions
In the past, research was conducted in the form of designing a structure on a computer, analyzing its characteristics, and summarizing the results in a paper. Recently, we have been using 3D printers to create intuitively designed structures and analyze their characteristics by actually touching them. In addition, I have more opportunities to present my research results not only in the form of papers, but also as concrete objects. I often receive unexpected ideas and stimulation by having people from different fields look at the actual molded structures, and this motivates me in my research. For example, I am focusing on applications to acoustic control and soft robotics using design methods based on the “force” and “form” approach to structures. Furthermore, I have also explored the potential for using deformable structures in educational toys, which has provided new possibilities for development.
I hope that my research will not just remain a challenge, but that its outcomes will extend beyond architecture and find practical applications in various real-world scenarios. I look forward to seeing how future architecture, design, and society will evolve through this work.
